Work
- Definition: A force of magnitude F acting on an object
that moves by a distance d, at an angle
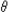 with
respect to the displacement of the object,
does a work W = Fd cos with
respect to the displacement of the object,
does a work W = Fd cos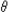 on
it. on
it.
- Sign: If W > 0 (W < 0) then
the force tends to accelerate (decelerate) the object's motion.
Kinetic Energy
- Definition: The energy an object has because of its motion,
KE = (1/2) mv2.
- Relationship with work: The net work done by all forces on
an object between two points equals the change in KE of the object
between those two points,
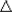 KE = Wnet. KE = Wnet.
Potential Energy
- Definition: The energy an object has because of its location
with respect to sources of force. A force can be used to define a potential
energy if it is conservative: the work it does between two points doesn't
depend on the path taken between those two points.
- For gravity near Earth's surface: The potential energy is
PEG = mgy, where y is the height from any reference point chosen
for a given problem.
- Elastic force: The force is given by Hooke's law, FS = –kx,
where k is the
spring's stiffness constant, and the potential energy is PES =
(1/2) kx2.
Conservation of Energy
- Most general statement: The total amount of energy in the
universe remains constant.
- Individual object: Its energy is the sum E = KE + PE + Einternal,
and it can change when it interacts with other objects and its environment,
for example because of work done on the object (or heat added to it).
- Mechanical energy: The sum Emech = KE + PE.
If all the forces that do work on an object are
conservative, then the object's mechanical
energy
remains the same. This can be written
as KE + PE = constant, or
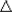 KE + KE + 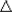 PE =
0, or E1 = E2.
Otherwise, if some forces are non-conservative and/or heat is added, PE =
0, or E1 = E2.
Otherwise, if some forces are non-conservative and/or heat is added, 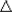 KE + KE + 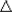 PE =
Wother + Q, or E1 +
Wother + Q = E2. PE =
Wother + Q, or E1 +
Wother + Q = E2.
Power
- Definition: The average power produced/used/transmitted over
a time
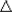 t is
P = t is
P = 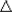 E/ E/ t. t.
|